Curve sketching calculus
Calculus is a subset of mathematics concerned with the study of continuous transition. First fundamental theorem of calculus PDF 20 Second fundamental theorem PDF 21 Applications to logarithms and geometry PDF - 14 MB 22 Volumes by disks and shells.

Graph Sketching Graph Sketch Graphing Plotting Points
How to get those points.

. Unit 6 - Implicit. We have so far integrated over intervals areas and volumes with single double and triple integrals. How to solve optimization problems.
Differential equations Sketching slope fields. Tangent lines and linear approximation. A plane simple closed curve is also called a Jordan curveIt is also defined as a non-self-intersecting continuous loop in the plane.
The inner radius in this case is the distance from the y-axis to the inner curve while the outer radius is the distance from the y-axis to the outer curve. Asymptotes and Other Things to Look For. Curve sketching PDF - 18 MB 11 Max-min problems PDF - 11 MB 12 Related rates PDF - 10 MB 13 Newtons method and other applications.
Calculus is also known as infinitesimal calculus or infinite calculus. We now investigate integration over or along a curveline integrals are really curve integrals. Comparing a Function and its Derivatives.
We will give some general guidelines for sketching the plot of a function. Logarithm Opens a modal Analyzing a function with its derivative Opens a modal Quiz 3. Back in the day curve sketching by hand was an important part of precalculusBut with the advent of the graphing calculator sketching curves by hand isnt usually necessary any more.
Version 2 Covers all topics for the AP Calculus AB exam but was built for a 90-minute class that meets every other dayThis course was built BEFORE the current Course and Exam Description from CollegeBoard but covers all the same material. Substantial portions of the content examples and diagrams have been redeveloped with additional contributions provided by experienced and practicing instructors. Approximation and Curve Sketching Part B.
Printable in convenient PDF format. Topics include parametric polar and vector functions and series. The prerequisites are high school or college algebra geometry and trigonometry.
Early Transcendentals originally by D. This example will also illustrate why this method is usually not the best. Guided step-by-step explanations to your math solutions.
6 to 30 characters long. Sketching graphs of functions and their derivatives. This calculus course covers differentiation and integration of functions of one variable and concludes with a brief discussion of infinite series.
Powers of sine and cosine. Buy our solution manual here. Lessons and packets are longer because they cover more material.
Sketching a parametric curve is not always an easy thing to do. Curve sketching with calculus. What about the length of any curve.
Roots y-axis-intercept maximum and minimum turning points inflection points. Level up on the above skills and collect up to 400 Mastery points Start quiz. Differential equations Reasoning.
Implicit Differentiation and Inverse Functions. Must contain at least 4 different symbols. Some Properties of Integrals.
Approximations Maxima. It was built for a 45-minute class period that meets every day so the lessons are shorter than our Calculus Version 2. The Fundamental Theorem of Calculus.
Pre-Algebra Algebra Pre-Calculus Calculus Linear Algebra math help. Optimization Related Rates and Newtons Method Part C. The cross-sectional area is then.
Mean Value Theorem Antiderivatives and Differential Equa Exam 2 3. The book is designed for students in engineering physics mathematics chemistry and other sciences. Determining the length of a planar curve using a definite integral.
Problem Solving. The Definite Integral. The first derivative test.
Explore the concepts methods and applications of differential and integral calculus. The second derivative test. Curve sketching is a calculation to find all the characteristic points of a function eg.
Learn integral calculus for freeindefinite integrals Riemann sums definite integrals application problems and more. This book covers calculus of a single variable. 8 Techniques of Integration.
Approximation and Curve Sketching Part B. The course below covers all topics for the AP Calculus AB exam but was built for a 90-minute class that meets every other day. Lets take a look at an example to see one way of sketching a parametric curve.
Free Calculus worksheets created with Infinite Calculus. Note that this is not always a correct analogy but it is useful initially to help visualize just what a parametric curve is. Both of these are then x distances and so are given by the equations of the curves as shown above.
Definition and Basic Rules Part B. Connecting a function its first derivative and its second derivative. We use the language of calculus to describe graphs of functions.
Graphing calculators are allowed on most calculus exams even AP Calculus so you can graph your function on the TI-89 to get an idea of the overall shape. Increasing and decreasing functions. Is there a way to make sense out of the idea of adding infinitely many infinitely small things.
Riemann Sum Tables. The Jordan curve theorem states that the set complement in a plane of a Jordan curve consists of two connected components that is the curve divides the plane in two non-intersecting regions that are both connected. Points of inflection.
Motion Along a Line. 58 Sketching Graphs of Functions and Their Derivatives 59 Connecting a Function Its First Derivative and Its. Two young mathematicians discuss the novel idea of the slope of a curve.
Unit 5 - Curve Sketching 51 Extrema on an Interval 52 First Derivative Test 53 Second Derivative Test Review - Unit 5. Unit 0 - Calc Prerequisites Summer Work 01 Summer Packet. By a Single Polar Curve 99 Finding the.
Optimization Related Rates and Newtons. Area Under a Curve by Limit of Sums. Support us and buy the Calculus workbook with all the packets in one nice spiral bound book.
Concavity and inflection points. This approachable text provides a comprehensive understanding of the necessary techniques. As with other integrals a geometric example may be easiest to understand.
Relative and absolute maximum and minimum points. Breakdown of the steps and substeps to each solution. Just in a different order.
The integral is the measure of the region under the curve while the derivative is the measure of the rate of change of a function. It is suitable for a year-long or two-semester course normally known as Calculus I and II in the United States. Slope of a curve at a point.
The Calculus CLEP exam is approximately 60 limits and differential calculus and 40 integral calculus. ASCII characters only characters found on a standard US keyboard. Calculus is fundamental to many scientific disciplines including physics engineering and economics.
Guichard has been redesigned by the Lyryx editorial team. Available online 247 even at 3AM Cancel subscription anytime. Ability to take a photo of your math problem using the app.

How To Sketch Level Curves Vector Calculus Vector Calculus Calculus Math Notes

Identifying And Matching The Graphs Of First Second Derivatives F F F In 2022 Ap Calculus Calculus Graphing

Pin On Calculus

Pin On Calculus

Pin On Calculus Lesson Ideas

Which One Doesn T Belong Limits Ap Calculus Ap Calculus Calculus Math Puns

Pin On Calculus
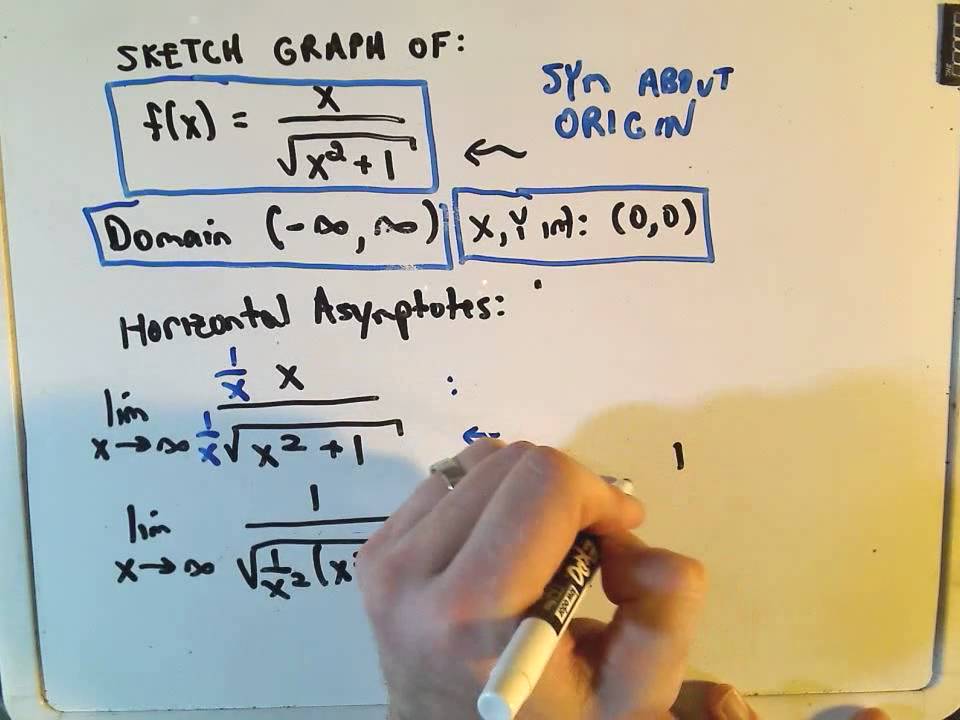
Pin On Calculus

6 1a Slope Fields Calculus Calculus Physics And Mathematics Mathematics

Calculus Curve Sketching 1st 2nd Derivative Tests Flip Book

Pin On Calculus

Pin On College Advice

Wodb For 1st Derivative Test Apcalculus Calculus Ap Calculus Calculus Math Puns

Curve Sketching

Pin On Calculus 1

Pin On Give Me A Sine

Ap Calculus Curve Sketching Tips Tricks